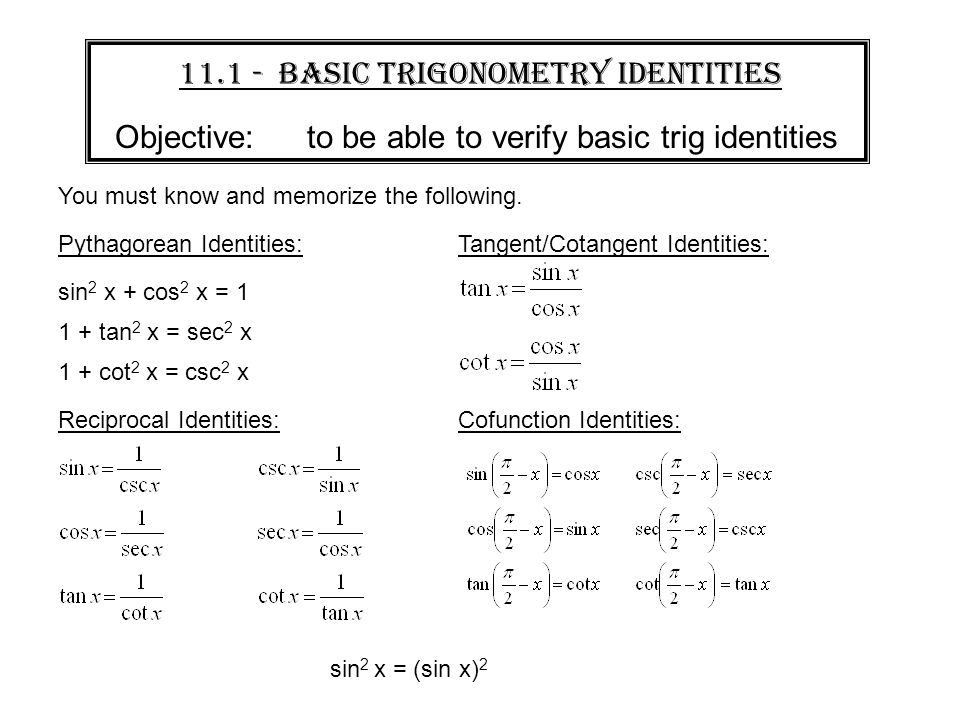
110 Prosthaphaeresis identities 1101 Proof of sine identities;1 cos A But sinA cosA =tanA and 1 cosA =secA (Notethat thedefinitionofthesecantofA is 1 cosA) Hence tan2 A1=sec2 A Thisisanotherimportantidentity Key Point tan2 A1=sec2 A Onceagain,returningto sin 2Acos A =1 wecandividethroughbysin2 A togive sin2 A sin 2A cos2 A sin A = 1 sin2 A But cosA sinA =cotA and 1 sinA =cosecA$\sec^2{x}\tan^2{x} \,=\, 1$ $\sec^2{A}\tan^2{A} \,=\, 1$ Remember, the angle of a right triangle can be represented by any symbol but the relationship between secant and tan functions must be written in that symbol Proof Learn how to prove the Pythagorean identity of secant and tan functions in mathematical form by geometrical method

Summary Of Trigonometric Identities
Tan^2 + 1 identity
Tan^2 + 1 identity-Pythagorean identities are identities in trigonometry that are extensions of the Pythagorean theorem The fundamental identity states that for any angle1102 Proof of cosine identities;



Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download
Various identities and properties essential in trigonometry Mathematics reference Trigonometric identities 18 Ma 2 MathRef Various identities and properties essential in trigonometry Legend x and y are independent variables, d is the differential operator, int is the integration operator, C is the constant of integration Identities tan x = sin x/cos x equation 1 cot x = cos x/sin xTrigonometric Identities Pythagoras's theorem sin2 cos2 = 1 (1) 1 cot2 = cosec2 (2) tan2 1 = sec2 (3) Note that (2) = (1)=sin 2 and (3) = (1)=cos Compoundangle formulae cos(A B) = cosAcosB sinAsinB (4) cos(A B) = cosAcosB sinAsinB (5) sin(A B) = sinAcosB cosAsinB (6) sin(A B) = sinAcosB cosAsinB (7) tan(A B) = tanA tanB 1 tanAtanB (8) tan(A B) = tanA tanB 1Verify the Identity (1sin(x)^2)(1tan(x)^2)=1 Start on the left side Apply Pythagorean identity Tap for more steps Apply pythagorean identity Rearrange terms Apply pythagorean identity Convert to sines and cosines Tap for more steps Apply the reciprocal identity to Apply the product rule to Simplify Tap for more steps One to any power is one Cancel the common
Sin2 Acos 2A = 1 sec A = 1tan2 A cosec2A = 1cot2A 2 3 Using the identities to solve equations 4 wwwmathcentreacuk 1 c mathcentre 09 1 Introduction In this unit we are going to look at trigonometric identities and how to use them to solve trigonometric equations A trigonometric equation is an equation that involves a trigonometric function or functions When we solve aFree math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantly25 Proof of Compositions of trig and inverse trig
$\tan^2{\theta} \,=\, \sec^2{\theta}1$ The square of tan function equals to the subtraction of one from the square of secant function is called the tan squared formula It is also called as the square of tan function identity Introduction The tangent functions are often involved in trigonometric expressions and equations in square form TheThere is no answer available Request an answerHow do you use the fundamental identities to prove other identities?



Proof Tan 2 1 Sec 2 Youtube



Solved Establish The Identity 1 Tan 2 Theta 1 Tan 2 Chegg Com
The denominator 1 tan^2(x) = = = (2) 3 When you divide the numerator (expression (1)) by the denominator (expression (2)), cos^2(x) cancels, and the remaining expression is (2) 3 When you divide the numerator (expression (1)) by the denominator (expression (2)), cos^2(x) cancels, and the remaining expression is , which you can rewrite = =Sin 2 (x) cos 2 (x) = 1 tan 2 (x) 1 = sec 2 (x) cot 2 (x) 1 = csc 2 (x) sin(x y) = sin x cos y cos x sin y cos(x y) = cos x cosy sin x sin y · As an example, we will verify that the equation \\tan^{2}(x) 1 = \sec^{2}(x)\ is an identity A proper format for this kind of argument is to choose one side of the equation and apply existing identities that we already know to transform the chosen side into the remaining side It usually makes life easier to begin with the more complicated looking side (if there is one) In our


What Is The Formula Of Tan2x Quora



Powers Of Trigonometric Functions
Cos2A = (1 Tan 2 A) / (1 Tan 2 A) Sin 2 A = (1 Cos2A) / 2 Cos 2 A = (1 Cos2A) / 2 Half Angle Identities Solving Word Problems Using Trigonometric Identities Step 1 Understanding the question and drawing the appropriate diagram are the two most important things to be done in solving word problems in trigonometry Step 2 If it is possible, we have to split the givenThis is one of the Pythagorean identities In the same way, we can derive two other Pythagorean identities 1tan 2 θ = sec 2 θ;Identities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify Statistics Arithmetic Mean Geometric Mean Quadratic Mean Median Mode Order Minimum Maximum Probability MidRange Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf



Cofunction Identities In Trigonometry With Proof And Examples Owlcation
Calculus Precalculus Mathematics for Calculus 6th Edition Verify the identity cos 2 x − tan 2 x sin 2 x = cot 2 x − sec 2 x more_vert Verify the identity cos 2 x − tan 2 x sin 2 x = cot 2 x − sec 2 x Buy Find launch Precalculus Mathematics for Calcu 6th Edition Stewart 5 others Publisher Cengage Learning ISBN Buy Find launch Precalculus MathematicsIt is seen that `sec^4 x tan^4 x = 1 tan^2 x` is not an identity, instead `sec^4x tan^4x = 1 2*tan^2x` Approved by eNotes Editorial Team We'll help your grades soar Start your 48hourThe key Pythagorean Trigonometric identity are sin 2 (t) cos 2 (t) = 1 tan 2 (t) 1 = sec 2 (t) 1 cot 2 (t) = csc 2 (t) So, from this recipe, we can infer the equations for different capacities additionally Learn more about Pythagoras Trig Identities Dividing through by c 2 gives a 2/ c 2 b 2/ c 2 = c 2/ c 2 This can be simplified


Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero



Solution Prove The Identity Cos X 1 Sin X Cos X 1 Sin X 2 1 Tan 2 X Sec X
2 Identities involving calculus 21 Preliminaries;Account Details Login Options Account Management Settings · Proving the identity $(\tan^2(x)1)(\cos^2(x)1)=\tan^2(x)$ Ask Question Asked 2 years, 1 month ago Active 2 years, 1 month ago Viewed 65 times 1 $\begingroup$ Proving the trigonometric identity $(\tan{^2x}1)(\cos{^2(x)}1)=\tan{^2x}$ has been quite the challenge I have so far attempted using simply the basic trigonometric identities based on the Pythagorean
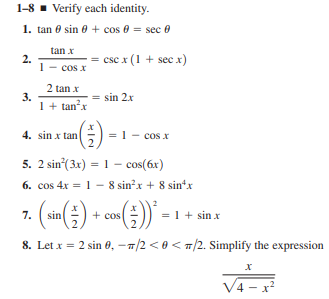


Answered 1 8 Verify Each Identity 1 Tan 0 Bartleby


Quandaries Queries Math Central
22 Sine and angle ratio identity; · Sine, tangent, cotangent and cosecant in mathematics an identity is an equation that is always true Meanwhile trigonometric identities are equations that involve trigonometric functions that are always true This identities mostly refer23 Cosine and angle ratio identity;
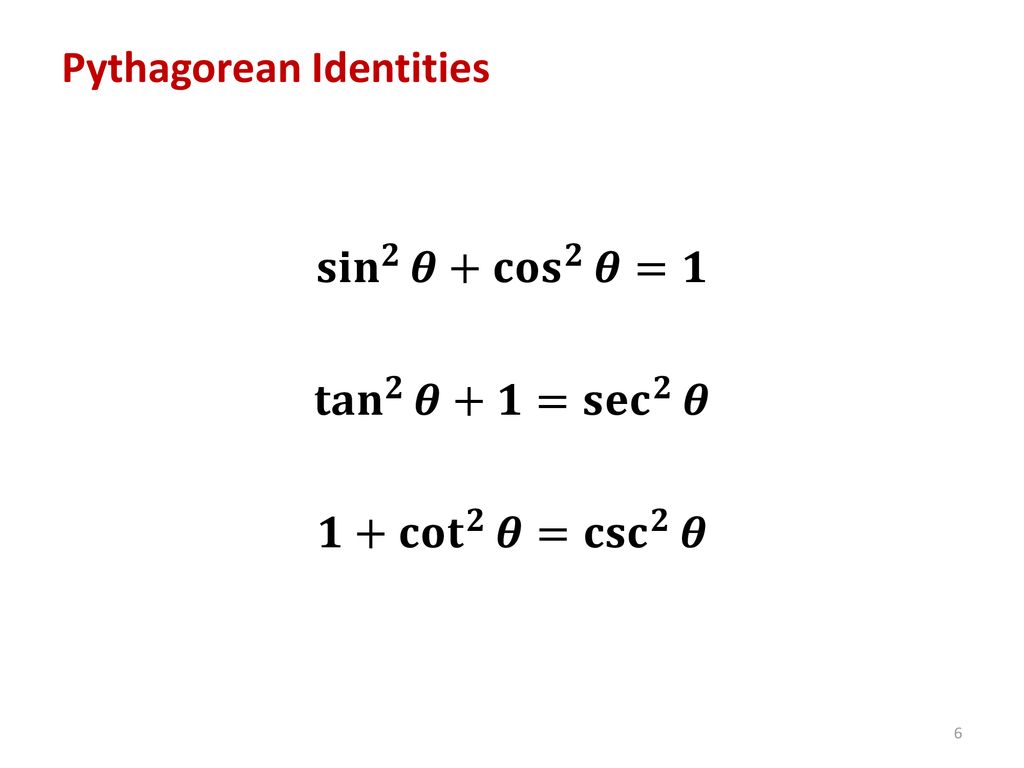


5 1 5 2 Trigonometric Identities Ppt Download



1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
Tanx = t Sec^2 xdx= dt So now it is, 1/ (1t)^2dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx Integral of the function 1tanxcos2x https//mathstackexchangecom/q/ Let I = ∫ 1tanxcos2xNotebook Groups Cheat Sheets;19 Miscellaneous the triple cotangent identity;


How Can One Prove That Math Tan 2x 2 Cot X Tan 2x Cot 2 X Math Quora
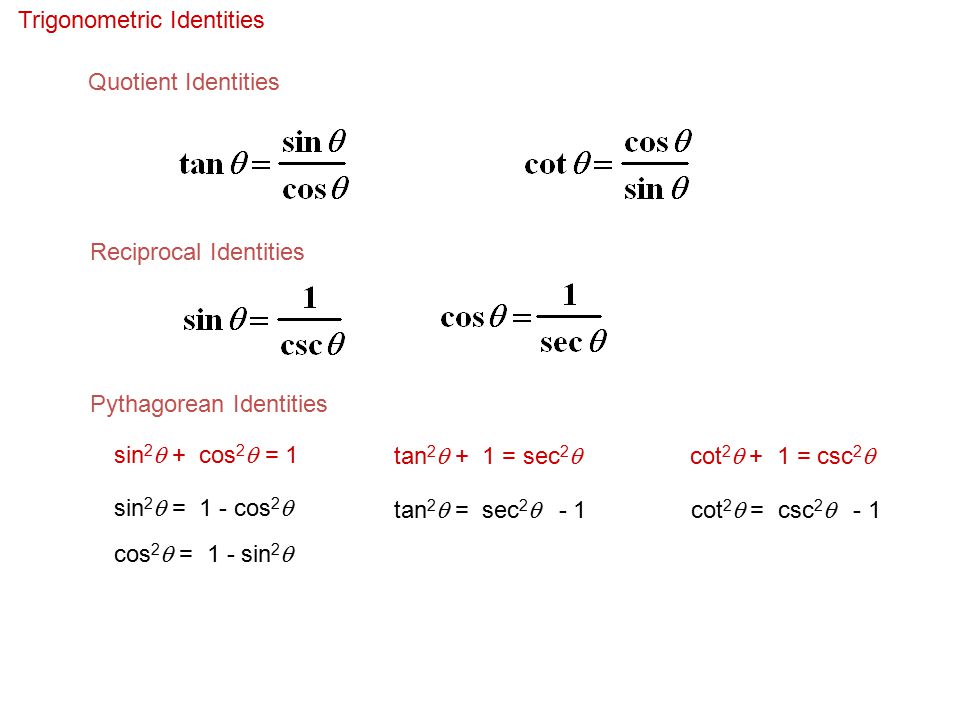


11 Basic Trigonometric Identities An Identity Is An Equation That Is True For All Defined Values Of A Variable We Are Going To Use The Identities To Ppt Download
Yes, sec 2 x−1=tan 2 x is an identity sec 2 −1=tan 2 x Let us derive the equation We know the identity sin 2 (x)cos 2 (x)=1 ——(i) Dividing throughout the equation by cos 2 (x) We get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) We know that sin 2 (x)/cos 2 (x)= tan 2 (x), and cos 2 (x)/cos 2 (x) = 1 So the equation (i) after substituting becomes tan 2 (x) 1= 1Anyways I looked at the solutions manual and they magic out $$1 \tan x \tan 2x = 1\tan x\left(\frac{2 \tan x}{1\tan ^2x}\right) $$ which I recognize as the double angle forumla sort of, I just don't understand why I can use that and how they magiced it into this It is just too difficult to think of an equation in an equation in an equation I tried working with this and got nowhere near\(\sin^{2}x\cos^{2}x=1\) \(\tan^{2}x1=\sec^{2}x\) \(\cot^{2}x1=\csc^{2}x\) Examples Example 1



Using Trigonometric Identities Video Khan Academy


Prove Sin2x 2tanx 1 Tan 2x Socratic
· Prove this identity Tan^2(0)/1 tan^2(0) = sin^2(0) Note i placed a bracket around t 154 Views Prove this identity Tan^2(0)/1 tan^2(0) = sin^2(0) Note i placed a bracket around the zeros because i didnt want to look like it is to the power of And the zero is supposed to be the symbol theta Answer Use the trigonometric To see more answers head over to CollegeTo integrate tan^22x, also written as ∫tan 2 2x dx, tan squared 2x, (tan2x)^2, and tan^2(2x), we start by utilising standard trig identities to change the form of the integral Our goal is to have sec 2 2x in the new form because there is a standard integration solution for that in formula booklets that we can use We recall the Pythagorean trig identity, and multiply the angles by 2We can start with the identity sin 2 (x)cos 2 (x)=1 If we divide through the equation by cos 2 (x), we get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) If we look at the left hand side of the equation sin 2 (x)/cos 2 (x) is equal to tan 2 (x), and cos 2 (x)/cos 2 (x) is equal to 1 (as it is divided through by itself), the left hand side becomes tan 2 (x) 1 Now if we look at the



Trig Identities And Formulas Pre Calculus Quiz Quizizz
.JPG)


Every Day I M Calculatin I D3 Unit Q Pythagorean Identities
Verify the Identity 1/(1sin(y)^2)=1tan(y)^2 Start on the right side Apply Pythagorean identity Tap for more steps Rearrange terms Apply pythagorean identity Convert to sines and cosines Tap for more steps Apply the reciprocal identity to Simplify Tap for more steps Apply the product rule to One to any power is one Apply Pythagorean identity in reverse Because theJoin for Free Problem Show that the identity $$ 1\cot ^{2} \theta=\csc Uh oh!The half‐angle identity for tangent can be written in three different forms In the first form, the sign is determined by the quadrant in which the angle α/2 is located Example 5 Verify the identity Example 6 Verify the identity tan (α/2) = (1 − cos α)/sin α Example 7 Verify the identity tan (α − 2) = sin π/(1 cos α)



Verify The Trigonometric Identity 1 1 Sin 2 X 1 Tan 2 X In 21 Identity Sins Tan
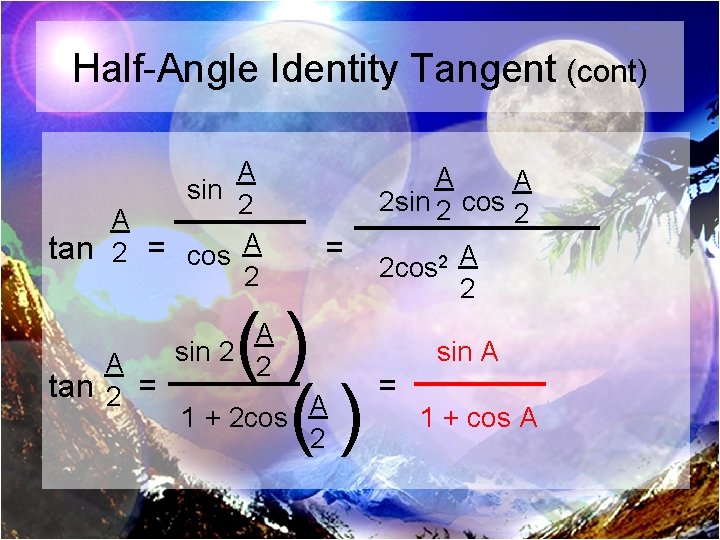


Chapter 5 Trigonometric Identities Section 5 1 Fundamental
Simply note that the following identity holds tan 2 x = 1 − t a n 2 x 2 t a n x which can be easily checked by the following tan 2 x = c o s 2 x s i n 2 x sin 2 x = 2 sin x cos x cos 2 x = cos 2 x − sin 2 xThese identities are known collectively as the tangent halfangle formulae because of the definition of (cos φ, sin φ) on the unit circle, draw the line passing through it and the point (−1, 0) This point crosses the yaxis at some point y = t One can show using simple geometry that t = tan(φ/2) The equation for the drawn line is y = (1 x)t The equation for the intersection of24 Cosine and square of angle ratio identity;



Which Of The Following Four S See How To Solve It At Qanda
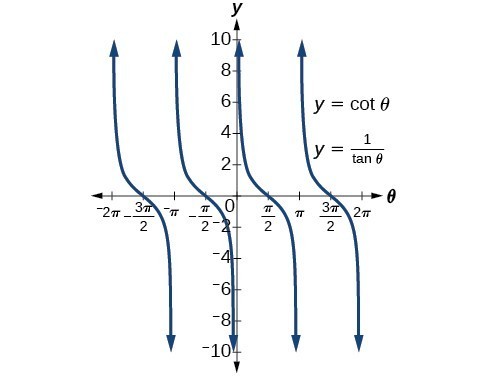


Solving Trigonometric Equations With Identities Precalculus Ii
· Get an answer for 'Proving trigonometric identities Prove sin2A = 2tanA/1 tan^2A' and find homework help for other Math questions at eNotes · How do you apply the fundamental identities to values of #theta# and show that they are true? · Conditional trigonometrical identities We have certain trigonometric identities Like sin 2 θ cos 2 θ = 1 and 1 tan 2 θ = sec 2 θ etc Such identities are identities in the sense that they hold for all value of the angles which satisfy the given condition among them and they are called conditional identities



How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic



Ppt Analytic Trig Powerpoint Presentation Free Download Id
· This proves the third powerreducing identity, $\tan^2 \theta = \dfrac{1 – \cos \theta}{1 \cos \theta}$ We've just shown how we can derive the three powerreducing identities using a doubleangle formula It's also possible for us to actually verify this identity using the halfangle identity Let's go ahead and master the three identities by solving the problems shownFree trigonometric identity calculator verify trigonometric identities stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more Accept Solutions Graphing Practice;Ask for it or check my other videos and playlists!##### PLAYLISTS #####


Double Angle And Multiple Angle Identities


7 Proving Ids Trig Functions Identities
Start with the right side of the identity 48 sec4 x = 1 2 tan2 x tan4 x SOLUTION Start with the right side of the identity 49 sec2 x csc2 x = sec2 x csc2 x SOLUTION Start with the left side of the identity 50 ENVIRONMENT A biologist studying pollution situates a net across a river and positions instruments at two different stations on the river bank to collect samples In the1tan2 a = sec2 a As it is known that tan a is not defined for a = 90° therefore identity 2 obtained above is true for 0 ≤ A · A follow up proof to accompany sin^2 cos^2 =1 Another identity that is used quite a bit, especially in calculus involving trigonometric functions


Important Trigonometric Identiti


What Is The Identity That Validates Sinx 3cos X P 3 And Show That Tan X 6 9 3 23 Quora
1 Trigonometric identities An identity is a relation which is always true To emphasise this the symbol '≡' is often used rather than '=' For example, (x1)2 ≡ x2 2x1 (always true) but (x1)2 = 0 (only true for x = −1) Task (a) Using the exact values, evaluate sin2 θReciprocal Identities csc𝜃= 1 sin𝜃 sec𝜃= 1 cos𝜃 cot𝜃= 1 tan𝜃 Pythagorean Identities sin2𝜃cos2𝜃=1 tan 2𝜃1=sec2𝜃 1cot2𝜃=csc2𝜃 Sum & Difference Identities sin( )=sin cos cos sin sin ( − ) = sin cos − cos sin cos( )=cos cos −sin sin cos( − )=cos cos sin sin tan( )= tan tan 1−tan tan tan( − )= tan −tan 1tan tan Double–Angle IdentitShow that the identity 1\tan ^{2} \theta=\sec ^{2} \theta follows from \sin ^{2} \theta\cos ^{2} \theta=1 Join our free STEM summer bootcamps taught by experts Space is limitedRegister Here 🏕 Books;
x-1=sec(squared)x.jpg)


10 Identity Tan Squared X 1 Sec Squared X Trigonometry Educator Com


Prove The Identity Tan 3 X 1 Tan 2 X Cot 3 X 1 Cot 2 X 1 2sin 2 X Cos 2 X Sin X Cos X Sarthaks Econnect Largest Online Education Community
1cot 2 θ = cosec 2 θ;Didn't find what you were looking for?Complement and Supplement of a Ratio The complementary angles are a pair of two angles such that their sum is equal to 90° The complement of an angle θ is (90 θ) The trigonometric ratios of complementary angles are sin (90° θ



How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic



Trigonometry Identity Tan 2 X 1 Sec 2 X Youtube



Example 15 Prove That Sec 2 Theta 1 Tan 2 Theta Sin Theta Cos Theta 1 Sin Theta Cos Theta 1 Sec Theta Tan Theta Using The Identity



List Of Trigonometric Identities Wikipedia



Trigonometric Identities Simplify Expressions Video Lessons Examples And Solutions



Integration Calculus Notes



1 1 Tan 2 Theta 1 1 Cot 2 Theta 1 Sin 2 Theta Sin 4 Theta Brainly In
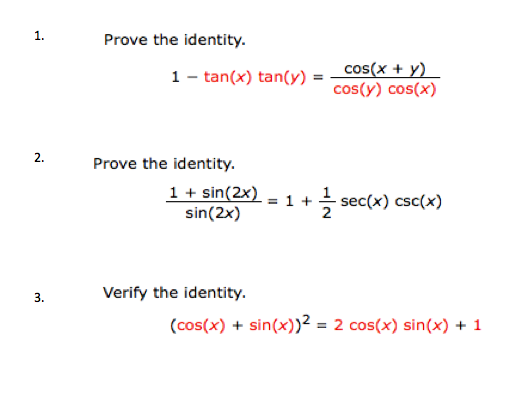


Solved Prove The Identity 1 Tan X Tan Y Cos X Y Chegg Com
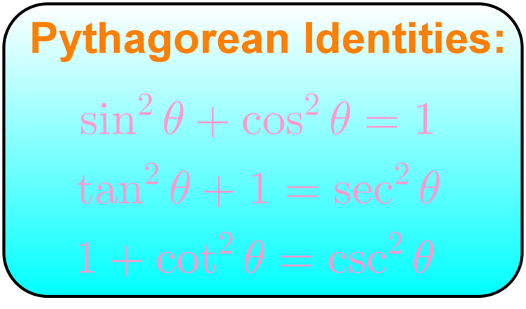


Understanding Pythagorean Identities Studypug



Precalculus Trigonometry Trig Identities 29 Of 57 Formula For Lowering Power Tan 2 X Youtube



Tangent Half Angle Formula Wikipedia



Prove The Identity 1 Tan 2x 1 Tan 2x 1 2sin 2x In Urdu Hindi Youtube



Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube



Solved Verify The Identity Tan 2 0 1 Cos 2 1 Tan Chegg Com
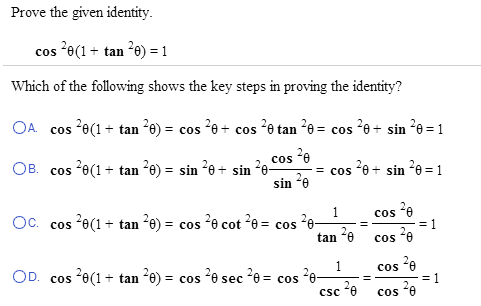


Solved Prove The Given Identity Cos 2 Theta 1 Tan2 Th Chegg Com



Tan2 1 Proove Sec Tan3cosec 2 3 2 Maths Trigonometric Identities Meritnation Com



1 Cosx 1 Cosx Tan 2x Secx 1 2prove Brainly In



Cos 2 1 Tan 2 1 Youtube


How I Remember Trig Identities Part 2 Beyond Solutions



Solved Verify The Identity 4 2 Tan X Secx2tan X 1 Whic Chegg Com



Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf



Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download



Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco



5 1 Fundamental Trig Identities Sin 1cos 1tan 1 Csc Sec Cot Csc 1sec 1cot 1 Sin Cos Ppt Download



Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco



Evaluating Trig Integral Int Tan 3x Sec 2x Dx Mathematics Stack Exchange



Integration Calculus Notes



How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic



Art Of Problem Solving
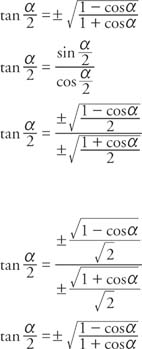


Tangent Identities


Worksheet On Trigonometric Identities Establishing Identities Hints


Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download


Trigonometric Functions Expressed By The Tangent Of The Half Angle Trigonometric Identities



Tangent Half Angle Formula Wikipedia



Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x



Prove The Following Identity Left Frac 1 Tan A 1 Cot A Right 2 Tan 2 A Angle A Is Acute
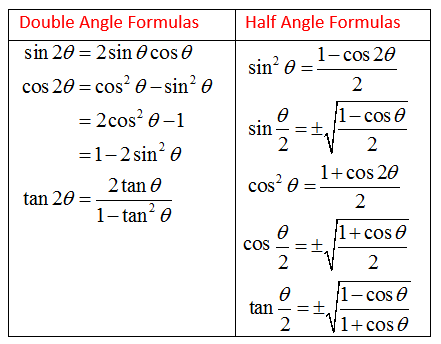


Double Angle Formula And Half Angle Formula Video Lessons Examples And Solutions
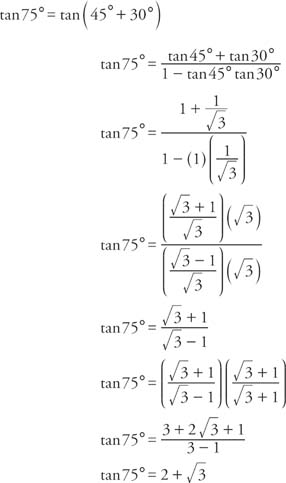


Tangent Identities


Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community



Pdf Revisited Arctan 1 Arctan 2 Arctan 3
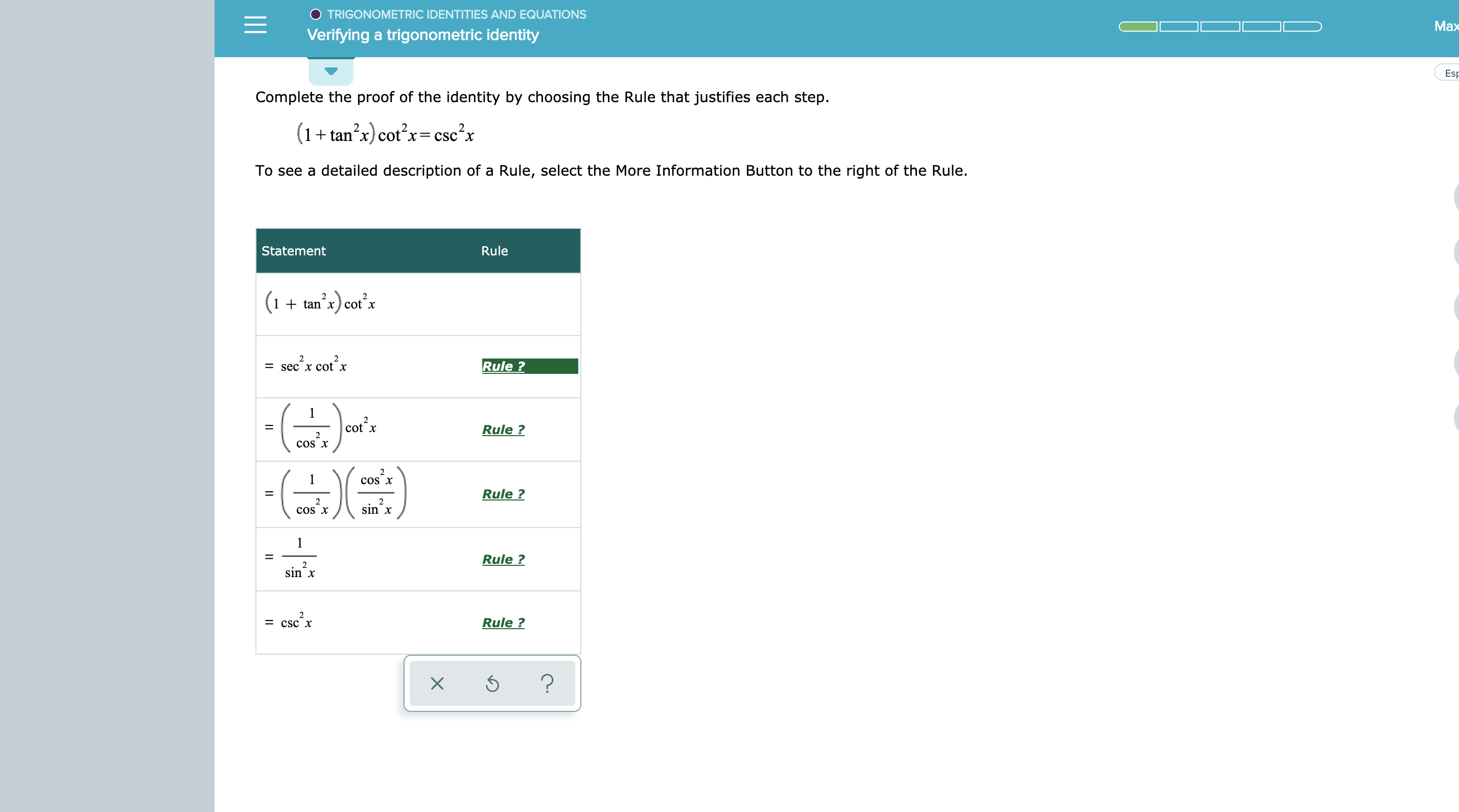


Answered Trigonometric Identities And Equations Bartleby



What Is The Value Of Sin 2theta 1 1 Tan 2theta



Pythagorean Trig Identities Recall Pythagoras Theorem Trig Identities
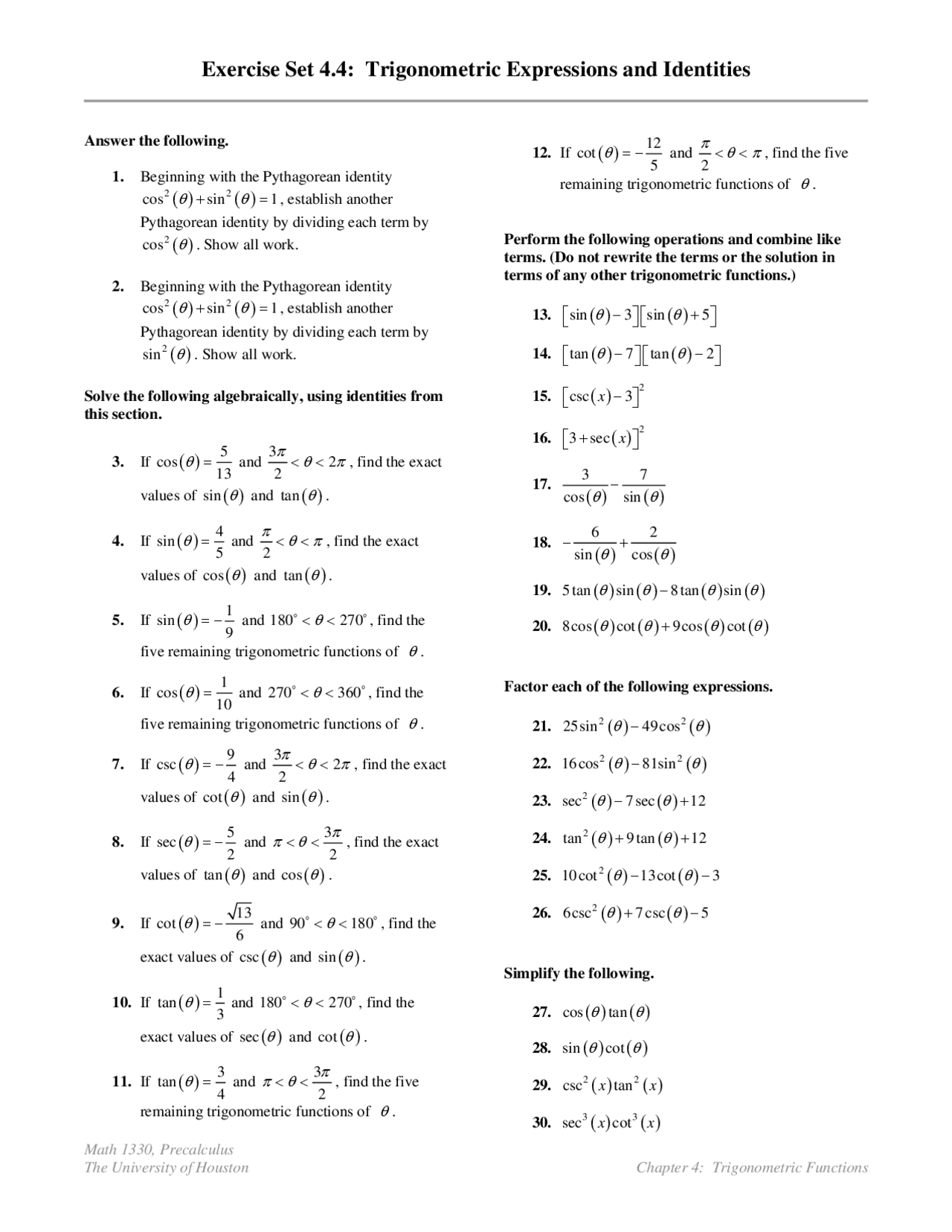


Exercise Set 4 4 Trigonometric Expressions And Identities Math 1330 Docsity



Check My Work Evaluating Tan Frac 7 Pi 8 Using A Half Angle Formula Mathematics Stack Exchange



Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download



Prove That 1 1 Tan 2 A 1 1 Cot 2 A 1 Sin
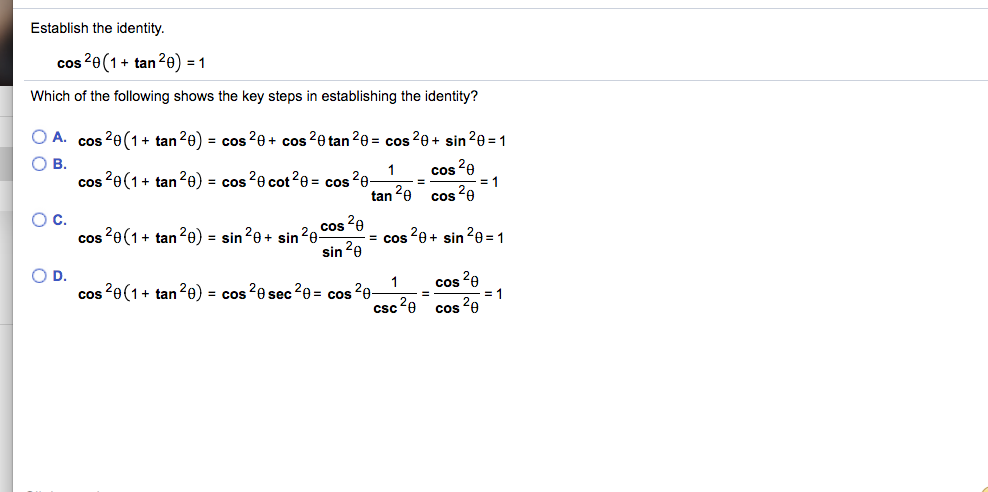


Solved Establish The Identity Cos 2 1 Tan 2 1 Which Chegg Com


View Question For Questions 1 5 Decide Whether The Equation Is A Trigonometric Identity Explain Your Reasoning



Verifying A Trigonometric Identity Cos X 1 Tan 2 X Cos X Sec X 1 Youtube
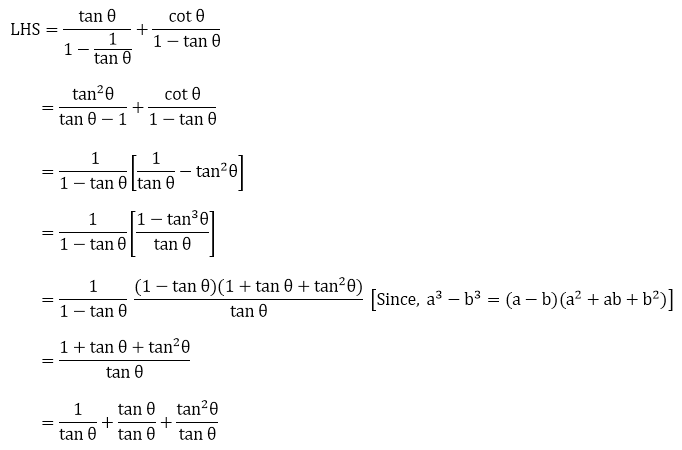


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf



Summary Of Trigonometric Identities



How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
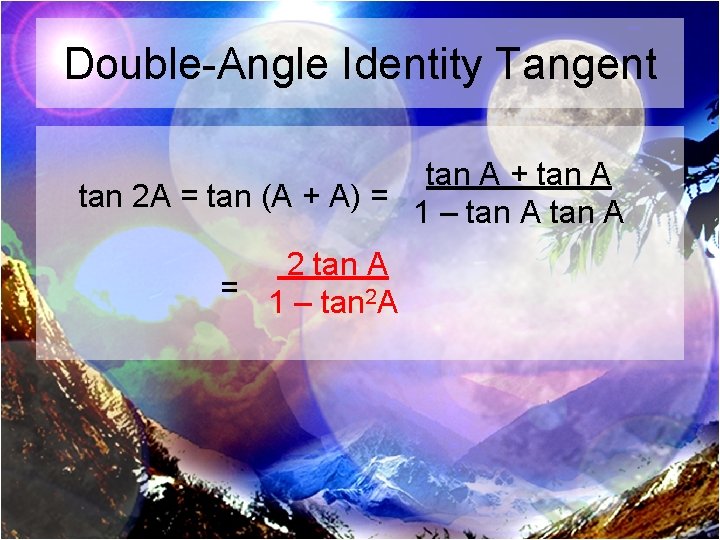


Chapter 5 Trigonometric Identities Section 5 1 Fundamental



Solved 2 Prove The Following Trig Identities A Prove Chegg Com
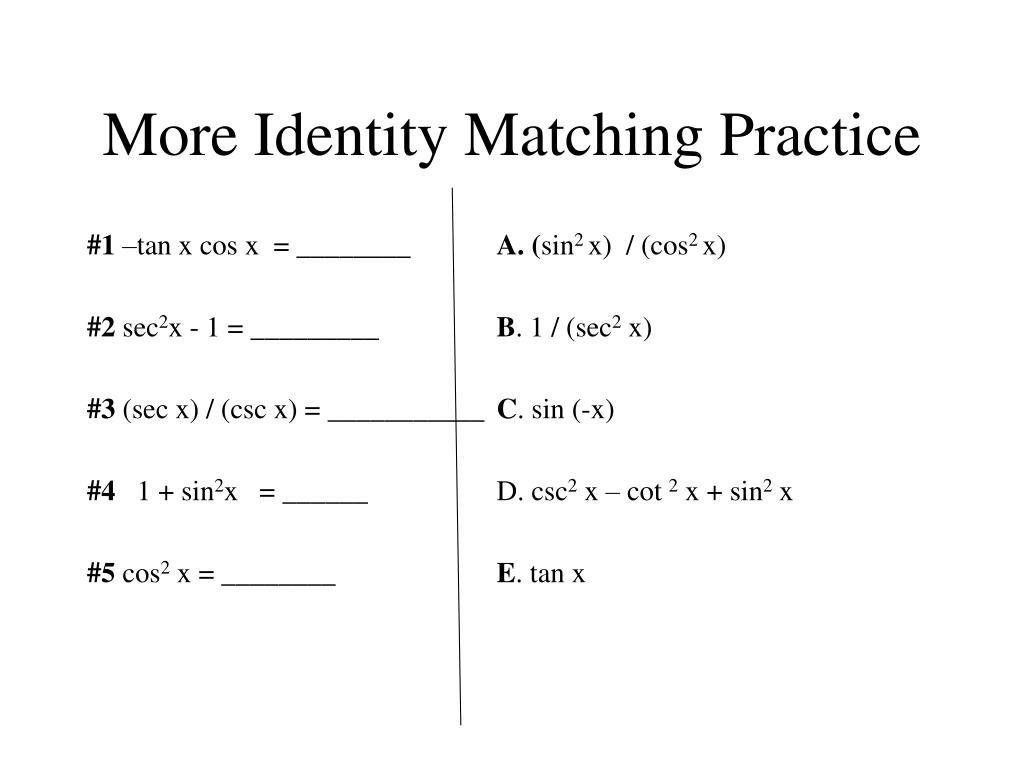


Ppt 5 1 Fundamental Trig Identities Powerpoint Presentation Free Download Id
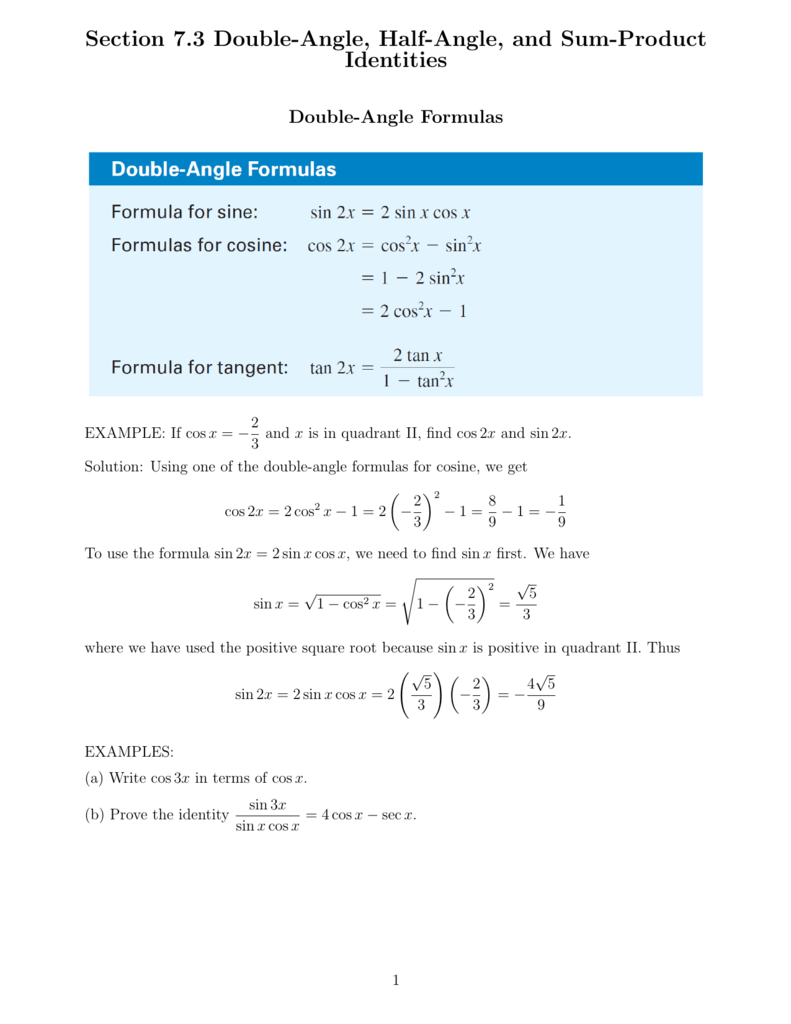


Section 7 3 Double Angle Half Angle And Sum


Cochranmath Solving Trigonometric Equations


Half Angle Identities



Double Angle Formulas Trigonometry Teachoo 2x 3x Formula Provi
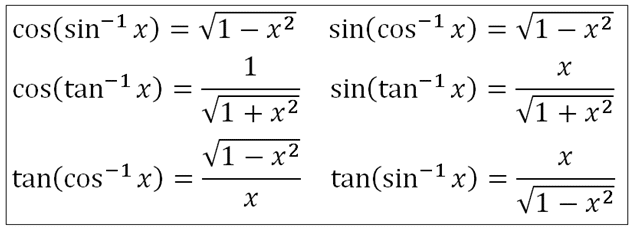


Inverse Trig Identities Reciprocal Of Trigonometric Function Trig



Cos2x Sin2x 1 1 Tan2x Sec2x Cot2x 1 Csc2x Cofunction



F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2


A Trig Identity
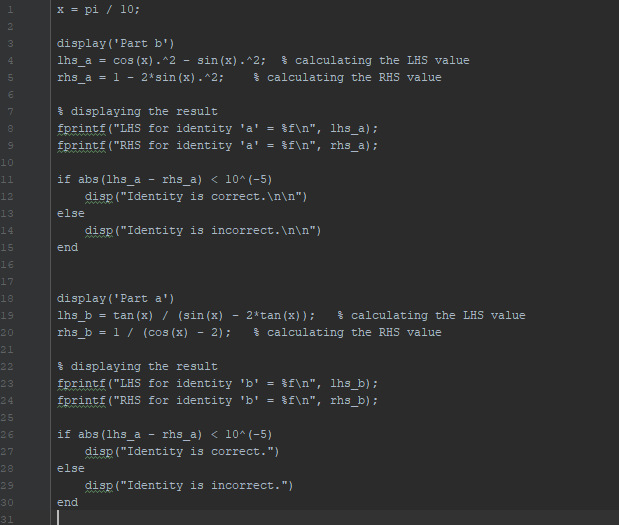


Solved Two Trigonometric Identities Given 1 Tan X 2 X Cos X Sin X 1 2sin1 B Sin X 2tan X Cos X 2 Q



Integrate Sec 2x Method 1
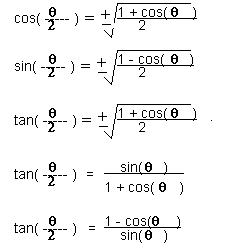


Double And Half Angle Formulas



Integrate Tan 2x



Summary Of Trigonometric Identities



0 件のコメント:
コメントを投稿